- Equations de Cauchy-Riemann
-
Équations de Cauchy-Riemann
Les équations de Cauchy-Riemann en analyse complexe, ainsi nommées en l'honneur d'Augustin Cauchy et Bernhard Riemann, sont deux équations aux dérivées partielles exprimant une condition nécessaire et suffisante pour qu'une fonction (d'une variable complexe, à valeurs complexes) différentiable au sens réel en un point soit différentiable au sens complexe en ce point.
En d'autres termes, ce sont les conditions à ajouter à la différentiabilité au sens réel pour obtenir la différentiabilité au sens complexe.
Lorsque la fonction est différentiable au sens réel en tout point d'un ouvert, ces équations expriment une condition nécessaire et suffisante pour qu'elle soit holomorphe sur cet ouvert.
On considère une fonction
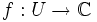 d'une variable complexe, définie sur un ouvert U du plan complexe
d'une variable complexe, définie sur un ouvert U du plan complexe  . On utilisera les notations suivantes :
. On utilisera les notations suivantes :- la variable complexe
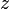 sera notée
sera notée 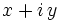 , où x, y sont réels
, où x, y sont réels - les parties réelle et imaginaire de
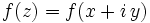 seront notées respectivement
seront notées respectivement 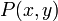 et
et 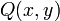 , c'est-à-dire :
, c'est-à-dire : 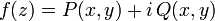 , où
, où 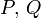 sont deux fonctions réelles de deux variables réelles.
sont deux fonctions réelles de deux variables réelles.
Sommaire
Fonctions
 -différentiables d'une variable complexe
-différentiables d'une variable complexeDéfinition
On dit que la fonction
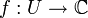 est différentiable au sens complexe, ou
est différentiable au sens complexe, ou  -différentiable (on dit encore dérivable) en un point
-différentiable (on dit encore dérivable) en un point 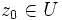 si la limite (finie)
si la limite (finie) 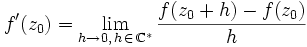 existe ; on l'appelle dérivée de f en
existe ; on l'appelle dérivée de f en 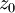 .
.Il est important de remarquer que la condition de
 -différentiabilité pour les fonctions de variable complexe est bien plus contraignante que la condition analogue pour les fonctions de variable réelle. La différence est la suivante :
-différentiabilité pour les fonctions de variable complexe est bien plus contraignante que la condition analogue pour les fonctions de variable réelle. La différence est la suivante :- dans
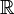 , il y a essentiellement deux manières de s'approcher d'un point : à droite, ou à gauche. Une fonction de variable réelle est dérivable en un point si et seulement si le "taux d'accroissement" admet en ce point une limite à droite et une limite à gauche ayant la même valeur (finie)
, il y a essentiellement deux manières de s'approcher d'un point : à droite, ou à gauche. Une fonction de variable réelle est dérivable en un point si et seulement si le "taux d'accroissement" admet en ce point une limite à droite et une limite à gauche ayant la même valeur (finie) - dans
 , il y a une infinité de manières de s'approcher d'un point ; chacune d'elles doit donner lieu à une limite (finie) du "taux d'accroissement", ces limites étant de plus toutes égales.
, il y a une infinité de manières de s'approcher d'un point ; chacune d'elles doit donner lieu à une limite (finie) du "taux d'accroissement", ces limites étant de plus toutes égales.
Un cas important
On dit qu'une fonction est holomorphe sur un ouvert de
 si elle est
si elle est  -différentiable en tout point de cet ouvert.
-différentiable en tout point de cet ouvert.Caractérisation des fonctions
 -différentiables en un point
-différentiables en un pointThéorème :
- Pour que la fonction f soit
 -différentiable en un point
-différentiable en un point  (où
(où 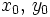 sont réels), il faut et il suffit :
sont réels), il faut et il suffit :
- qu'elle soit différentiable au sens réel en
- et que, de plus, elle vérifie les équations de Cauchy-Riemann en ce point. Ces équations peuvent s'écrire sous les formes équivalentes suivantes :
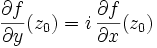
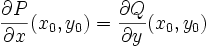 et
et 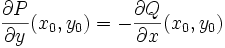
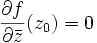
- qu'elle soit différentiable au sens réel en
- Dans ce cas :
- la différentielle de
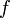 au point
au point 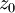 est l'application
est l'application 
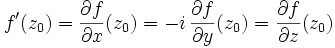
- la différentielle de
Démonstration du théorème- On garde les notations précédentes ; en particulier, on note r un réel tel que
 et
et  , et h un nombre complexe tel que
, et h un nombre complexe tel que 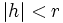 .
.
- On suppose que
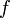 soit
soit  -différentiable en
-différentiable en 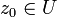 : alors
: alors 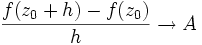 lorsque
lorsque  (on note
(on note 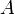 la dérivée
la dérivée 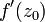 ).
).
- On définit
 (fonction d'une variable complexe):
(fonction d'une variable complexe):
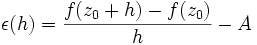 si
si 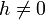 (*). Alors (par définition de A):
(*). Alors (par définition de A): 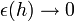 lorsque
lorsque 
- (*) peut s'écrire :
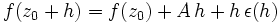 (pour
(pour  , et aussi pour
, et aussi pour 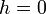 ),
), - ou encore :
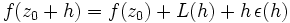 , où
, où 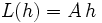 (**)
(**)
- Il est clair que l'application
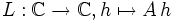 est
est 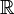 -linéaire (et même
-linéaire (et même  -linéaire, propriété plus forte). Par conséquent :
-linéaire, propriété plus forte). Par conséquent :
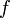 est
est 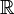 -différentiable en
-différentiable en 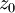
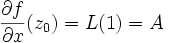 ,
, 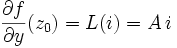 , ou :
, ou : 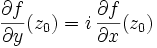 .
.
- On définit
- Réciproque : on suppose que
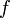 soit
soit 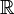 -différentiable en
-différentiable en 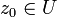 et que
et que 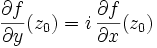 , autrement dit :
, autrement dit : 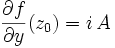 , où
, où 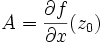 (contrairement à ce qu'on affirme souvent, on n'utilise ici aucune hypothèse de continuité des dérivées partielles : l'hypothèse précédente concerne un seul point ; il se pourrait que
(contrairement à ce qu'on affirme souvent, on n'utilise ici aucune hypothèse de continuité des dérivées partielles : l'hypothèse précédente concerne un seul point ; il se pourrait que 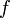 ne soit
ne soit 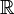 -différentiable qu'en ce point).
-différentiable qu'en ce point).
- Par hypothèse, en notant L la
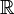 -différentielle de
-différentielle de 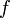 en
en 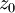 , on peut écrire :
, on peut écrire :
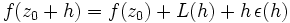 , où
, où 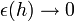 lorsque
lorsque 
- Si
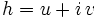 (u, v réels), alors, par
(u, v réels), alors, par  -linéarité de L,
-linéarité de L, 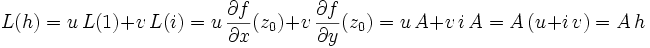
- Ainsi :
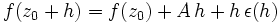 , et
, et 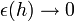 lorsque
lorsque 
- Si
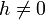 , on en déduit que :
, on en déduit que :  lorsque
lorsque  . L'existence de cette limite établit que
. L'existence de cette limite établit que 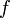 est
est  -différentiable en
-différentiable en 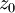 (c'est-à-dire :
(c'est-à-dire : 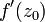 existe), et que
existe), et que 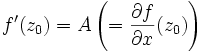 .
. - Ceci prouve aussi que lorsque
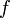 est
est  -différentiable en
-différentiable en 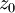 :
:
- sa différentielle est l'application
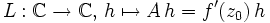 .
. 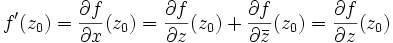 , car
, car 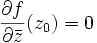 .
.
- sa différentielle est l'application
- Par hypothèse, en notant L la
- On suppose que
Un cas important
La caractérisation suivante des fonctions holomorphes est une conséquence immédiate du théorème précédent, appliqué en chaque point.
Théorème : une fonction
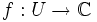 est holomorphe sur l'ouvert U de
est holomorphe sur l'ouvert U de  si et seulement si :
si et seulement si :- elle est
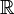 -différentiable en tout point de U,
-différentiable en tout point de U, - et elle vérifie les équations de Cauchy-Riemann en tout point de U
Remarque sur la continuité des dérivées partielles : on peut montrer (c'est un résultat important de la théorie de Cauchy) que toute fonction holomorphe sur un ouvert de
 y est analytique : cela signifie qu'au voisinage de chaque point, elle est développable en série entière ; donc, toute fonction holomorphe est indéfiniment dérivable, et a fortiori elle admet des dérivées partielles continues sur l'ouvert.
y est analytique : cela signifie qu'au voisinage de chaque point, elle est développable en série entière ; donc, toute fonction holomorphe est indéfiniment dérivable, et a fortiori elle admet des dérivées partielles continues sur l'ouvert.Exemples
- La fonction
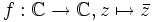 est de classe
est de classe 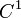 sur
sur  , donc elle y est
, donc elle y est 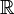 -différentiable ; mais elle n'est
-différentiable ; mais elle n'est  -différentiable en aucun point parce qu'elle ne vérifie nulle part les équations de Cauchy-Riemann. En effet, comme
-différentiable en aucun point parce qu'elle ne vérifie nulle part les équations de Cauchy-Riemann. En effet, comme 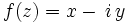 :
:
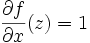 et
et 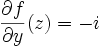
- ainsi, pour tout
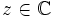 ,
, 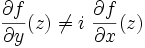 .
.
- La fonction
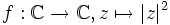 est de classe
est de classe 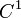 sur
sur  , donc elle y est
, donc elle y est 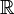 -différentiable ; elle est
-différentiable ; elle est  -différentiable en 0 et seulement en ce point (elle n'est holomorphe sur aucun ouvert, son ensemble
-différentiable en 0 et seulement en ce point (elle n'est holomorphe sur aucun ouvert, son ensemble  de
de  -différentiabilité étant d'intérieur vide).
-différentiabilité étant d'intérieur vide). - La fonction
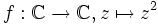 est holomorphe sur
est holomorphe sur  et pour tout
et pour tout 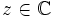 ,
, 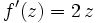 . En effet, si
. En effet, si 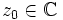 et
et 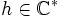 ,
, 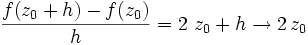 lorsque
lorsque 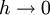 . On a
. On a 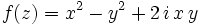 , donc :
, donc :
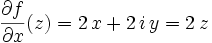
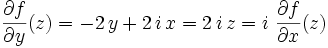 (équations de Cauchy-Riemann au point z)
(équations de Cauchy-Riemann au point z)
- Le caractère contraignant de la condition d'holomorphie est particulièrement saisissant quand on applique les conditions de Cauchy-Riemann à une fonction à valeurs réelles définie sur un ouvert de
 : les 2 dérivées partielles par rapport à x et à y doivent alors être nulles et la fonction doit être localement constante ! En d'autres termes, une fonction holomorphe réelle sur un ouvert connexe de
: les 2 dérivées partielles par rapport à x et à y doivent alors être nulles et la fonction doit être localement constante ! En d'autres termes, une fonction holomorphe réelle sur un ouvert connexe de  se réduit nécessairement à une constante.
se réduit nécessairement à une constante.
Par exemple, la fonction argument de z (réelle et non constante) n'est pas holomorphe. On vérifie d'ailleurs facilement que les équations de Cauchy-Riemann ne sont pas satisfaites, car ses dérivées partielles sont celles de arctan (y/x). Il en est évidemment de même de la fonction module de z (réelle et non constante).
- Portail des mathématiques
Catégories : Analyse complexe | Bernhard Riemann - la variable complexe
Wikimedia Foundation. 2010.
