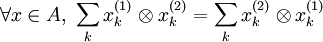Cocommutative
- Cocommutative
-
Cocommutativité
En mathématiques, la cocommutativité est une notion duale de la notion de commutativité.
Définition
Soit (A,Δ) une bialgèbre. Pour tout x appertenant à A, on note 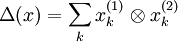 . La bialgèbre A est dite cocommutative si :
. La bialgèbre A est dite cocommutative si :
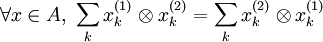
 Portail des mathématiques
Portail des mathématiques
Catégories : Algèbre | Groupes quantiques
Wikimedia Foundation.
2010.
Contenu soumis à la licence CC-BY-SA. Source : Article Cocommutative de Wikipédia en français (auteurs)
Regardez d'autres dictionnaires:
cocommutative — adjective Describing the relationship, in a coalgebra, that is the equivalent of an commutative one … Wiktionary
Formal group — In mathematics, a formal group law is (roughly speaking) a formal power series behaving as if it were the product of a Lie group. They were first defined in 1946 by S. Bochner. The term formal group sometimes means the same as formal group law,… … Wikipedia
Hopf algebra — In mathematics, a Hopf algebra, named after Heinz Hopf, is a structure that is simultaneously a (unital associative) algebra, a coalgebra, and has an antiautomorphism, with these structures compatible.Hopf algebras occur naturally in algebraic… … Wikipedia
Cofree coalgebra — In algebra, the cofree coalgebra of a vector space or module is a coalgebra analog of the free algebra of a vector space. The cofree coalgebra of any vector space over a field exists, though it is more complicated than one might expect by analogy … Wikipedia
Laurent polynomial — In mathematics, a Laurent polynomial in one variable over a ring R is a linear combination of positive and negative powers of the variable with coefficients in R . Laurent polynomials in X form a ring denoted R [ X , X −1] . They differ from… … Wikipedia
Coalgebra — In mathematics, coalgebras or cogebras are structures that are dual (in the sense of reversing arrows) to unital associative algebras. The axioms of unital associative algebras can be formulated in terms of commutative diagrams. Turning all… … Wikipedia
Quantum group — In mathematics and theoretical physics, quantum groups are certain noncommutative algebras that first appeared in the theory of quantum integrable systems, and which were then formalized by Vladimir Drinfel d and Michio Jimbo. There is no single … Wikipedia
Yetter-Drinfeld category — In mathematics a Yetter Drinfel d category is aspecial type of braided monoidal category.It consists of modules over a Hopf algebra which satisfy some additionalaxioms. Definition Let H be a Hopf algebra over a field k . Let Delta denote the… … Wikipedia
Group Hopf algebra — In mathematics, the group Hopf algebra of a given group is a certain construct related to the symmetries of group actions. Deformations of group Hopf algebras are foundational in the theory of quantum groups. DefinitionLet G be an arbitrary group … Wikipedia
Cocommutativité — En mathématiques, la cocommutativité est une notion duale de la notion de commutativité. Définition Soit (A,Δ) une coalgèbre. Pour tout x appartenant à A, on note La coalgèbre A est dite cocommutative si … Wikipédia en Français
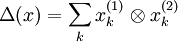 . La bialgèbre A est dite cocommutative si :
. La bialgèbre A est dite cocommutative si :